Superinsulator: Reversed Superconductor

one must also realize the significance of the observation” 

Ivar Giaever

Superconductivity, i. e. the ability of certain materials to carry loss-free current, appears due to the interaction of conducting electrons with the vibrational motion of atoms. As a result of the mediating action of atomic vibrations, electrons whose identical electric charges would ordinarily cause them to repel each other instead attract each other and combine into bound pairs called Cooper pairs. While individual electrons are fermions and cannot occupy the same quantum state, Cooper pairs are bosons. This means that at sufficiently low temperatures, Cooper pairs condense at the lowest possible quantum state to form a so-called Cooper condensate. To remove a Cooper pair from the condensate and turn it into two unbound electrons, one has to expend certain energy. The minimal energy required, E_min = 2Δ (where Δ is the superconducting gap), is the energy gap separating the ground quantum state from the states occupied by unbound electrons. The Cooper condensate is defined by a unique wave function spreading over the whole volume of a superconductor. When an applied current passes through a superconductor the condensate moves as a whole, with all the Cooper pairs remaining in the lowest energy state (the ground state).

In conventional conductors, the flow of electric current causes electrons to accelerate due to the presence of an applied electric field. As they accelerate, the electrons gain energy and climb to elevated energy levels. The energy accumulation cannot last indefinitely long. After a certain period, called the energy relaxation time, the electron loses its excess energy to the crystalline lattice of the conductor. This energy transfer from the electric field to the material creates electric resistance and causes thermal (Joule) losses. As a result, the conductor heats up.

In a superconductor the energy gap enables Cooper pairs to move with a constant velocity while remaining in the lowest energy state as long as their kinetic energy does not exceed E_min = 2Δ. In other words, electric current can flow through a superconductor without the application of an external electric field, i. e., without an expenditure of energy. For example, in a superconducting wire that has been shaped into a ring, current may persist forever. This reflects the absence of the electric resistance of a superconductor.

An excessively large current, at which the energy of an electron pair reaches 2Δ, destroys superconductivity. The same can be achieved via an appreciable increase of temperature and/or through the application of high magnetic fields. Thus, superconductivity is limited by maximal values of the current density, the temperature, and the magnetic field; these constraints are usually visualized as a phase diagram. The critical values of the current density, temperature, and magnetic field fully characterize a specific material.

Numerous experiments have demonstrated that critical temperatures are much lower for thin films of a material than for same material in bulk, and that thin enough films can even appear non-superconducting. Thus, in addition to the critical values of the current density, temperature, and magnetic field, we may note a critical thickness for the film. Moreover, it turns out that the effect of variation in film thickness is very different from variation in the three other parameters. Specifically, as film thickness decreases below its critical value, the film may not only become metallic (as one could have expected), but even insulating. The investigation of two-dimensional superconductivity and, particularly, of this unusual superconductor-to-insulator transition in films a few nanometers thick has become a key research direction in condensed matter physics in the past two decades.

In the mirror of low temperatures

At the Institute of Semiconductor Physics SB RAS, our investigations of the superconducting properties of thin titanium nitride films, prepared at the Interuniversity Microelectronic Center in Belgium, revealed that these films experience a sharp superconductor-insulator transition without an intermediate metallic phase. Films nearly identical in thickness and in resistance vs. temperature behavior above 0.5 K appear to choose unequivocally whether to turn superconductor or insulator at very low temperatures.

It is this, the so called critical region of the superconductor-insulator transition, which became the focus of our research at Regensburg University in Germany, where extremely low temperatures were available. Our main task was clarifying the nature of the insulating state of films that initially seemed likely to exhibit superconducting behavior. We soon found that these insulators have properties very different from those of ordinary insulators with an energy bandgap in their electronic spectra. For example, the width of the bandgap in our insulators turned out to depend strongly upon the applied magnetic field: first, it grew almost twice as the applied magnetic field increased from zero to some intermediate value, then dropped to almost zero upon further increase of the field. Eventually, in high magnetic fields the bandgap disappeared and the insulator transforms into a metal. Superconductors in the critical region also exhibited unusual behavior: even relatively weak magnetic fields drove superconductors into an insulating state, and further increase in the field transformed them into metals.

THE JOSEPHSON EFFECT AND THE JOSEPHSON JUNCTION NETWORK

The stationary Josephson effect consists in superconducting current flowing across a thin nonsuperconducting layer (such as dielectric, metal, or simply vacuum) separating two superconductors. This arrangement is called a Josephson junction. The terms are named after British physicist Brian David Josephson, who predicted the existence of the effect in 1962. This phenomenon was found experimentally by American physicists P. W. Anderson and J. M. Rowell in 1963.
Two-dimensional Josephson junction networks consisting of arrays of superconducting islands separated by thin nonsuperconducting layers are exemplary systems for the study of the superconductor-to-insulator transition. The properties of these networks are determined by two competing characteristic energies: E_J0, the energy of the Josephson coupling of adjacent islands, and E_C, the charging energy of a single Josephson junction, which is associated with the transfer of a 2e charge between neighboring islands. If E_J0 > E_C, the Josephson network is in its superconducting state, whereas if E_J0< E_C, the network is in its insulating state.
Note that both energies are determined by the material used and by the geometric size of the islands and the separations between them.
Importantly, the energy of the Josephson coupling can be additionally tuned by an applied magnetic field, which allows for observation of the magnetic field-driven superconductor-insulator transition as long as the parameters of the network have been properly chosen.
The figure illustrates this possibility. The red curve depicts the theoretical dependence of the Josephson coupling energy E_J on the magnetic field applied perpendicular to the plane of a square network. If the magnitude of E_C falls into range of the E_J variation, the system can be made to transit between the superconducting state corresponding to E_J > E_C and the insulating state corresponding to E_J < E_C

The behavior of the insulators under applied voltage was even more intriguing. At moderate temperatures the current was proportional to applied voltage in accordance with Ohm’s law. As temperatures were lowered, however, the dependence of current on applied voltage acquired a threshold character: there was no current as long as the applied voltage did not exceed some threshold value (in other words, the resistance of the film was infinite below some threshold voltage). Once the threshold voltage was exceeded, however, the current jumped by several orders of magnitude. Surprisingly, the current-voltage characteristics of our insulators and superconductors turned out to be mirror images of each other.

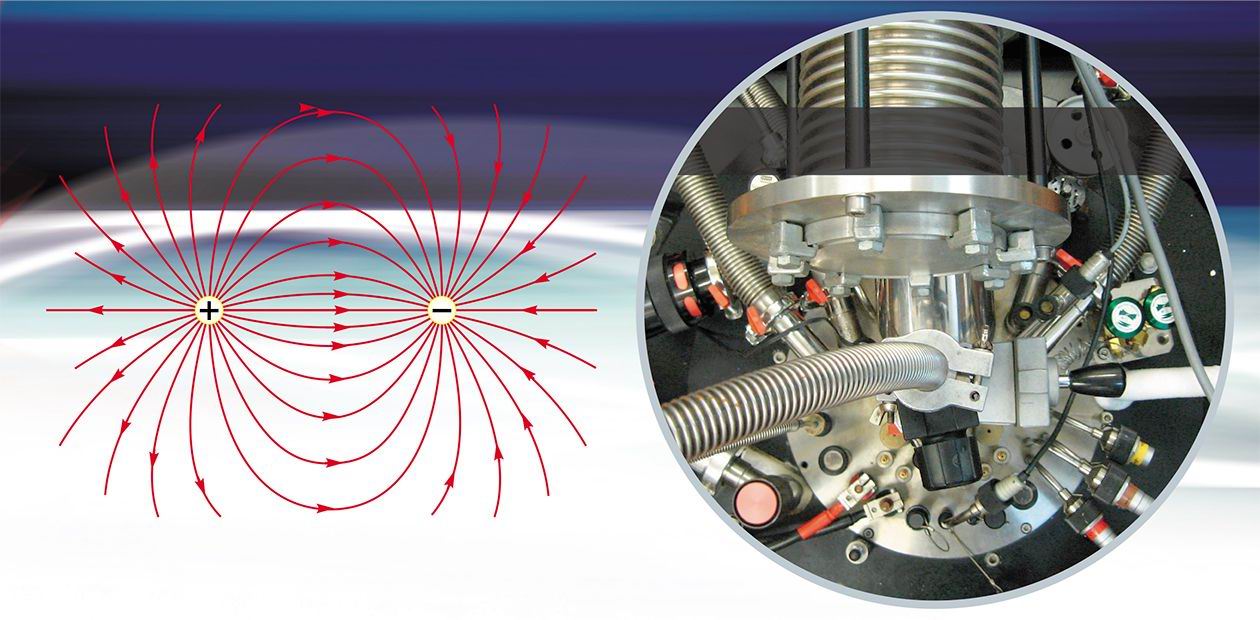
COULOMB INTERCATIONS IN THREE- AND TWO-DIMENSIONAL UNIVERSE

It is a fact of elementary physics that electric charges induce electric fields, which are vector quantities. These fields are commonly visualized using force lines that spring, by convention, from a positive charge and end at a negative one. The strength of the field is proportional to the density of these lines: the number of the lines piercing a square unit of area and perpendicular to the field is taken to be proportional to the electric field strength. If we consider a spherical cap over a fixed area, the number of force lines crossing its surface would decrease as 1/r², where r is the distance from the charge inducing the electric field. This spherical divergence of the force lines gives rise to the interaction energy between the charges (U), which decreases as 1/r.
The situation in the two-dimensional universe is dramatically different. Let us imagine that we constrict all the force lines to the plane. It is clear, then, that the density of the lines increases over what it was in the three-dimensional case. One can verify that in the two-dimensional world the strength of the electric field, i. e. the number of lines crossing a circular arc of fixed length, would decrease with distance as 1/r, which is much slower than its behavior in three dimensions. This implies that the interaction energy of two charges will now increase as charge separation grows according to the apparently counterintuitive relation U~ln(r). The main question, however, is whether such a situation can be realized in our three-dimensional world. The answer is “yes,” but some special conditions apply. For instance, the electric charge must be placed inside a film of high dielectric constant e; then, indeed, the electric force lines will be confined within the film up to the spatial scale r~ ed, and the two-dimensional Coulomb law and, accordingly, the logarithmic interaction between the charges holds within these distances. At larger spatial scales the electric force lines start to escape from the film and the usual three dimensional Coulomb behavior is restored. Hence, the energy necessary for dragging apart the charges of different sign to opposite edges of the film increases logarithmically with the film size as long as the latter does not exceed ed

All the accumulated research data, including data obtained via the scanning electron microscopy technique at temperatures down to 0.05 K, led us to conclude that in insulators that form within the critical region, the Cooper condensate survives in the form of isolated superconducting droplets (islands). We have called this insulator the Cooper-pair insulator. Thus, the critical films can be considered Josephson junction networks – a system of superconducting islands coupled via Josephson links. In the critical region, the system is in fragile equilibrium: even a tiny increase in the strength of the Josephson coupling resulting from a temperature decrease may effect the merging of independent superconducting islands into a superconducting ”continent,” which establishes a global superconductivity. On the other hand, if structural inhomogeneities get even slightly stronger, a temperature decrease will produce even more isolation among the islands, strengthening the insulating properties of the film. The notion of such a self-generated Josephson junction network explains perfectly the fan-like temperature dependences of the resistance – up and down routes taken by the thin films upon cooling – shown in the beginning of the article. But this is not the whole story.

Paradoxes of a two-dimensional universe

If the system of superconducting islands in a film is very near the threshold separating a superconductor from an insulator, the film develops a huge dielectric constant, the phenomenon known among specialists in two-phase systems as a “dielectric catastrophe.” As a result, all the electric fields induced by charges located inside the film become trapped there, while Coulomb interactions within the film acquire a two-dimensional character. That is, instead of the customary, three-dimensional Coulomb’s law in which the energy of the pair interaction decays inversely to the distance between the charges, the Coulomb interaction energy in the two-dimensional universe increases as the logarithm of the distance between the charges. The two-dimensionality of the Coulomb interaction explains why the energy gap we observed in our experiments on insulating films close to transition was so anomalously great. In order for a current to flow, an expenditure of energy necessary for separating charges of the opposite sign is needed: positive charges must move toward the negative electrode and vice versa. The amount of energy necessary for charge separation grows as the logarithm of the size of the system. An even more fascinating property of critical films is that the two dimensional charge plasma they confine experiences the Berezinskii-Kosterlitz-Thouless (BKT) transition: above the temperature of the transition, the positive and negative components of the charge plasma move independently, but below the transition the positive and negative charges bind into neutral dipoles that cannot move under an applied electric field. Separation of charges becomes practically impossible and the resistance of the film becomes infinite, making a Cooper pair insulator with exponentially large resistance into a superinsulator.

CURRENT FLOW THROUGH AN ARRAY OF SUPERCONDUCTING ISLANDS IMMERSED INTO AN INSULATING MEDIUM

Consider the flow of current across a system comprised of a voltage source, connecting wires, and a film with leads, where we view the film as an array of separated superconducting islands. Assume that the condition E_J < E_C  is satisfied and that the temperature of the experiment is below the charge Berezinskii-Kosterlitz-Thouless transition temperature, meaning that all the charges of opposite sign are bound into dipoles. Note that the system remains electrically neutral at all times. The propagation of positive charge from the voltage source towards the film is therefore accompanied by opposing motion of negative charge inside the film and, simultaneously, by the motion of negative charge from the source towards the film at the opposite side of sample. This kind of carrier transfer happens when bound pairs of positive and negative charges unbind and are dragged to opposite edges of the film, where they are annihilated by their mirror images in the metallic leads. Because of the two-dimensional character of the Coulomb interaction, the energy needed for the ultimate separation of each pair is proportional to the logarithm of the size of the film and can be shown to be equal to Δ_c= E_C ln(L/a), where L is the length of the film and a is the size of the elemental unit of the array. The quantum-mechanical formula describing the tunneling current reads I~exp (-Δ_c/W), where W represents the energy lost by the charge carrier during tunneling between two islands. Without these energy losses, commonly called the energy relaxation process, tunneling would be impossible because the energy levels of electrons vary between islands. In arrays of islands, energy relaxation occurs via the creation of the electron-hole pairs, and W is proportional to their density. At temperatures above the temperature of the Berezinskii-Kosterlitz-Thouless transition (T_BKT), W=k_BT (k_B is the Boltzmann constant). At temperatures below T_BKT, electrons and holes are bound together in pairs in two-dimensional systems, and the quantity W becomes exponentially large, reflecting the fact that creation of “free” unbound pairs capable of efficiently absorbing and emitting energy of any magnitude is practically impossible. This is the microscopic mechanism of the suppression of tunneling current in the superinsulating state

Thus, the superinsulator is actually the mirror image of a superconductor. Since the two-dimensional character of Coulomb behavior of the critical film is caused by the presence of superconducting islands, the superinsulator is destroyed at temperatures and magnetic fields above critical ones. The critical (threshold) voltage for a superinsulator is the analogue of the critical current for a superconductor. The domain in which a superinsulator can exist occupies the region in a phase diagram dual to that of a superconductor; similarly, the current-voltage characteristics of a superinsulator and a superconductor are mirror images of each other. In a superinsulating state Joule losses are absent since the current there is completely blocked. In this respect superinsulators are similar to superconductors where Joule losses are absent because of the absence of the voltage drop.

We thus arrived at the remarkable conclusion that Cooper pairing can give rise not only to superconductivity, but also to its opposite: the superinsulating state.

References
Edelman, V. S., Near the absolute zero, Moscow, Fizmatlit, 2001.
Kaganov, M. I. and Lifshitz, I. M., Quasiparticles, Moscow, Nauka, 1989.
Baturina T. I., Strunk C., Baklanov M. R. et al. Quantum Metallicity on the High-Field Side of the Superconductor-Insulator transition // Phys. Rev. Lett. 2007. V. 98, 127003.
Baturina T. I., Mironov A. Yu., Vinokur V. M. et al. Localized Superconductivity in the Quantum-Critical Region of the Disorder-Driven Superconductor-Insulator Transition in TiN Thin Films // Phys. Rev. Lett. 2007. V. 99, 257003.
Vinokur V. M., Baturina T. I., Fistul M. V. et al. Superinsulator and quantum synchronization // Nature. 2008. № 452, P. 613—615.
Sacépé B., Chapelier C., Baturina T. I. et al. Disorder-Induced Inhomogeneities of the Superconducting State Close to the Superconductor-Insulator Transition, // Phys. Rev. Lett. 2008. V. 101, 157006.
Chtchelkatchev N. M., Vinokur V. M., Baturina T. I. Hierarchical energy relaxation in mesoscopic tunnel junctions: Effect of a nonequilibrium environment on low-temperature transport // Phys. Rev. Lett. 2009. V. 103, 247003.